Fourier series
Answer
- The questioner was satisfied with and accepted the answer, or
- The answer was evaluated as being 100% correct by the judge.
1 Attachment
Erdos
-
May I ask why substitute I into k for the step with (**)? And how we can know that k=1 since pi/2 is used?
-
For any other k the integrals are zeroby formula (*).
-
Indeed integrals are all zero except gor k=1.
-
for k=1
-
So the integral shouldn't be zero?
-
You have infinite integrals. For each k you have an integral, and all of them are zero except the integral corresponding to k=1.
-
Is it true that for part(i), if k not equals to zero, then the required integral is -16/3?
-
No, your question doesn't make sense. The infinite sum start at k=1. So k=0 doesn't make much sense.
-
Sorry it's a typo. I mean for k not equals to 1. For part(i), if k not equals to 1, then is it true that the required integral is -16/3? Because your answer only consider the case when k=1.
-
Yes, we compute the integral of -8/3 separately. This can be viewed as the term corresponding for k=0. So there are two non-zero terms.
-
So do I only need to consider the case where k=1 in the solution? Or should I add another condition where k not equals to 1?
-
Yes, that's what I have done in the solution.
-
Alright. Thank you so much for your help!
-
The bounty was $8 and I will receive only $6.4 after commission of the website. I have spend more than 30 minutes to write a solution and about 15 minutes to answer your questions here. This is not really worth my time. Judging from your question it seems that you need someone to explain the fundamentals of these problems in a very detailed manner. You may want to create a new post, and ask your remaining questions there.
-
I'll consider your suggestion. Sorry for wasting your time but I really appreciate your help. Thank you so much.
-
I would be more than happy to help you understand these concepts, but working 45 minutes for $6 is not fair and I don't feel good about doing so! I decided to answer your question as I thought no one else will.
- answered
- 794 views
- $8.00
Related Questions
- Find the limit as x --> +inf
- Obtaining the absolute velocity of a moving train based on angle of raindrops with respect to vertical axis
- Evaluate$\int \sqrt{\tan x}dx$
- Equations of Motion and Partial Fractions
- Show that the line integral $ \oint_C y z d x + x z d y + x y d z$ is zero along any closed contour C .
- [Help Application of Integration]Question
- Evaluate $\int \sin x \sqrt{1+\cos x} dx$
- Solution to Stewart Calculus
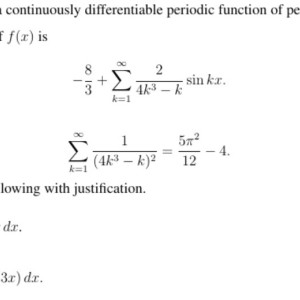
This is a time consuming problem. The bounty is too low.
How about just part(i)?