Calculus: INFINITE SERIES
Answer
Answers can only be viewed under the following conditions:
- The questioner was satisfied with and accepted the answer, or
- The answer was evaluated as being 100% correct by the judge.
3 Attachments
 Kav10
Kav10
2.1K
The answer is accepted.
Join Matchmaticians Affiliate Marketing
Program to earn up to a 50% commission on every question that your affiliated users ask or answer.
- answered
- 937 views
- $40.00
Related Questions
- Explain what the problem means in laymens terms.
- Finding only one real root for a function
- Please answer the attached question about Riemann integrals
- Find the volume of the solid obtained by rotating $y=x^2$ about y-axis, between $x=1$ and $x=2$, using the shell method.
- Please help me with this math problem I am struggling!
- Calculus 1
- Find $\lim\limits _{n\rightarrow \infty} n^2 \prod\limits_{k=1}^{n} (\frac{1}{k^2}+\frac{1}{n^2})^{\frac{1}{n}}$
- Improper integral
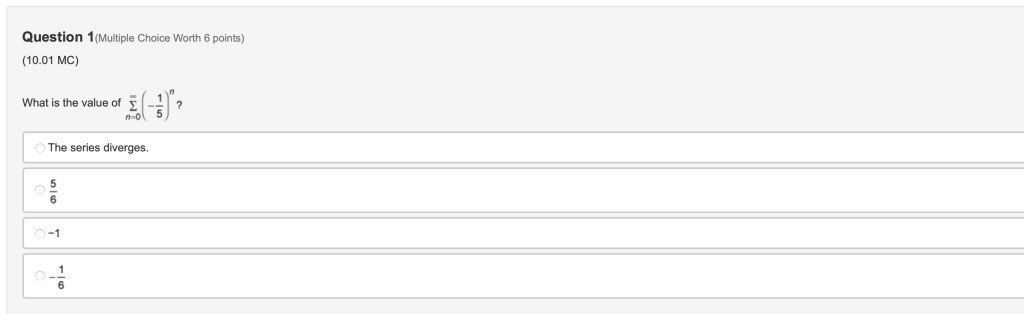
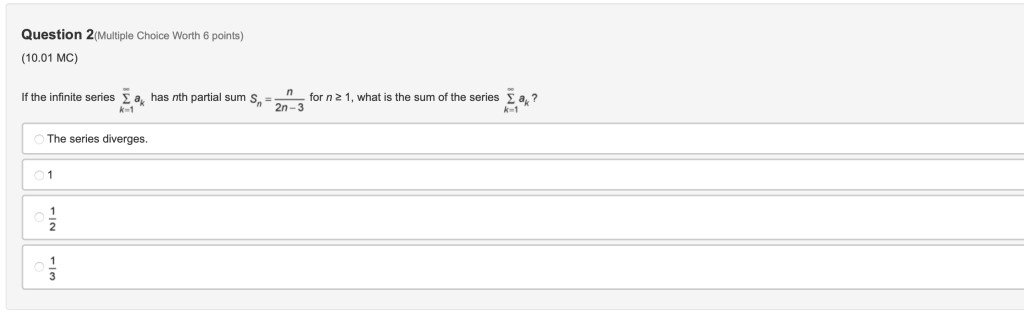
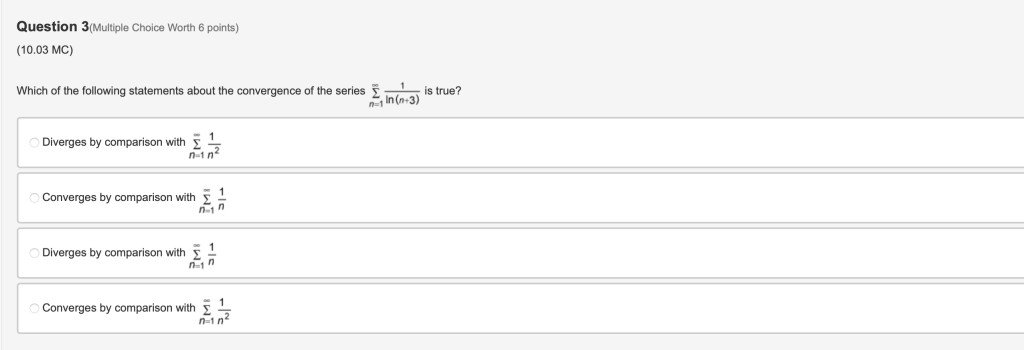
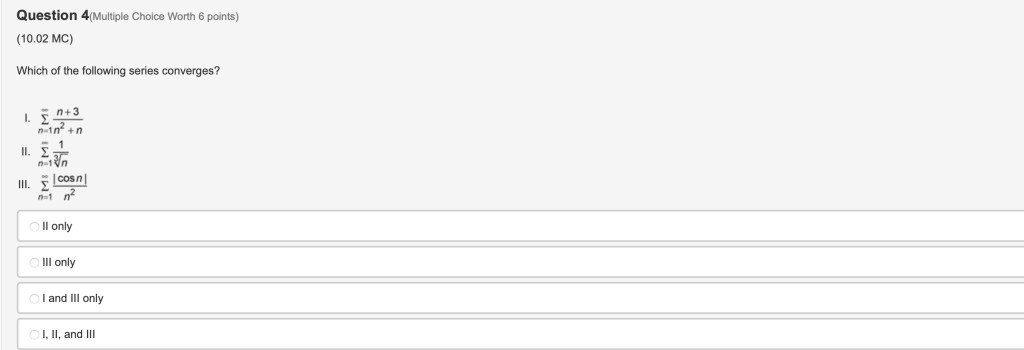
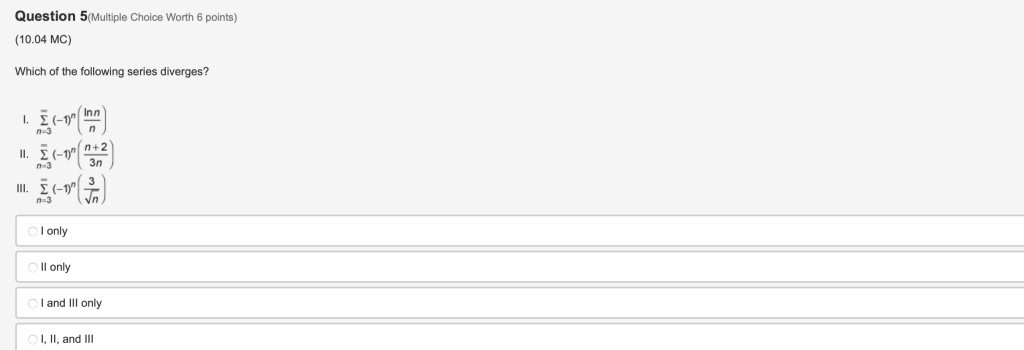

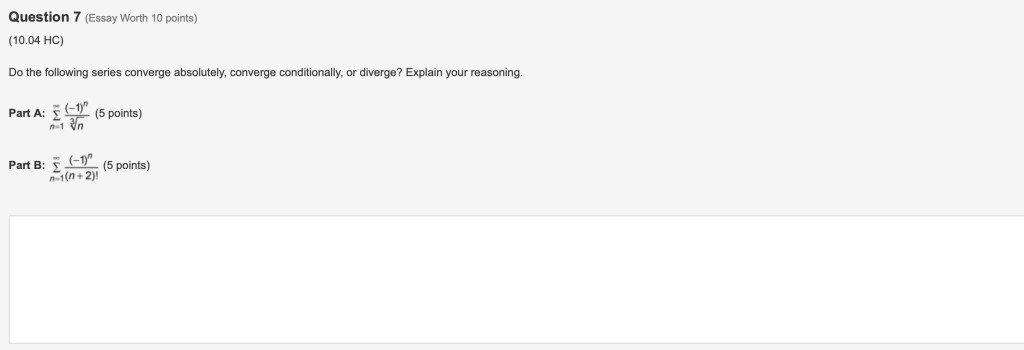

You can answer whenever you want; I will have three projects Tomorrow (Sunday) if you wanna answers (high bounty).
Yes, got it. Thanks.
YOU'RE AWESOME
Sure. What time tomorrow?
around 2 o'clock
Pacific or Eastern?
EDT, so eastern
Sounds good. I am in the west, so 11 am my time.
For the MCQ Above, give me the answer option to check if mine matches. And for the written, just be organized as always! THANKS
Sure. NO PROBLEM.