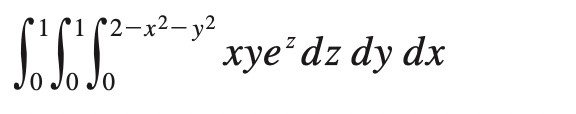Custom Solutions to Stewart Calculus, Integral
Answer
Sure, you came to the right place to get custom solutions given by experts.
For this integral we start by integrating with respect to $z$ : $$ \int _0 ^1 \int _0 ^1 \int_0 ^{2-x^2-y^2} xy e^{z}\,dz\,dy\,dx = \int _0 ^1 \int _0 ^1 xy e^{z}\,\Big]_0 ^{2-x^2-y^2}\,dy\,dx \\ = \int _0 ^1 \int _0 ^1 xy( e^{2-x^2-y^2}-1)\,dy\,dx. $$
Then we do the $y$ integral. For this let $u=2-x^2-y^2$ to get $du=-2ydy$ (remember that in the $y$ integral we treat $x$ as a constant!)
$$ \int _0 ^1 \int _0 ^1 xy( e^{2-x^2-y^2}-1)\,dy\,dx \\ \; \\ =\int _0 ^1 \int _0 ^1 \frac{-1}{2}x (e^{u}-1)\,du\,dx = \int _0 ^1 \frac{-1}{2}x (e^{u}-u)\,\Big]_0 ^1\,du\,dx \\ \; \\ =\int _0 ^1 \frac{-1}{2}x (e-2)\,dx = \frac{-(e-2)}{2}\,\frac{x^2}{2}\,\Big]_0 ^1 = \frac{-(e-2)}{4}$$
- accepted
- 1198 views
- $15.00
Related Questions
- Beginner Question on Integral Calculus
- Write a Proof
- Some final thoughts and closing out this question
- Is $\int_0^{\infty}\frac{x+3}{x^2+\cos x}$ convergent?
- Integral of $\arctan x$
- Find $lim_{x \rightarrow 0^+} x^{\ln x}$
- Integrate $\int x^2\sin^{-1}\left ( \frac{\sqrt{a^2-x^2} }{b} \right ) dx$
- Differentiate $\int_{\sin x}^{\ln x} e^{\tan t}dt$