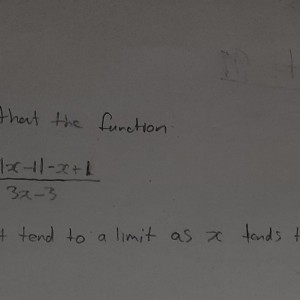Prove the Function
Answer
Answers can only be viewed under the following conditions:
- The questioner was satisfied with and accepted the answer, or
- The answer was evaluated as being 100% correct by the judge.
 Dynkin
Dynkin
779
The answer is accepted.
Join Matchmaticians Affiliate Marketing
Program to earn up to a 50% commission on every question that your affiliated users ask or answer.
- answered
- 1347 views
- $5.00
Related Questions
- Subspace of a Normed Linear Space
- Banach fixed-point theorem and the map $Tf(x)=\int_0^x f(s)ds $ on $C[0,1]$
- A constrained variational problem
- H is a Hilber space
- [ Banach Fixt Point Theorem ] $\frac{dy} {dx} = xy, \text{with} \ \ y(0) = 3,$
- Prove that $p_B :\prod_{\alpha \in A} X_\alpha \to \prod_{\alpha \in B} X_\alpha$ is a continuous map
- Two exercises in complex analysis
- Prove that every compact Hausdorff space is normal