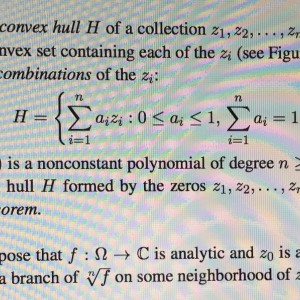
Two exercises in complex analysis
I am stuck on the attched two problems. I have no idea how to approach them.
Answer
Answers can only be viewed under the following conditions:
- The questioner was satisfied with and accepted the answer, or
- The answer was evaluated as being 100% correct by the judge.
1 Attachment
4.8K
The answer is accepted.
Join Matchmaticians Affiliate Marketing
Program to earn up to a 50% commission on every question that your affiliated users ask or answer.
- answered
- 1399 views
- $8.00
Related Questions
- Prove the Function
- Evluate $\int_{|z|=3}\frac{1}{z^5(z^2+z+1)}\ dz$
- Rouche’s Theorem applied to the complex valued function $f(z) = z^6 + \cos z$
- Some final thoughts and closing out this question
- Prove that $p_B :\prod_{\alpha \in A} X_\alpha \to \prod_{\alpha \in B} X_\alpha$ is a continuous map
- Uniform convergence of functions
- Let $(X, ||\cdot||)$ be a normed space. Let $\{x_n\}$ and $\{y_n\}$ be two Cauchy sequences in X. Show that the seqience Show that the sequence $λ_n = ||x_n − y_n|| $ converges.
- Prove Holder-continuity for $\mu_\lambda (x) = \sum\limits_{n=1}^\infty \frac{ \cos(2^n x)}{2^{n \lambda} }$