Prove that a reduced Gorenstein ring of Krull dimension 1 is not a complete intersection ring.
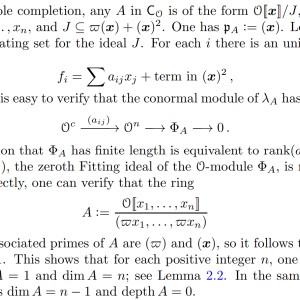
Let $A$ be a commutative, Noetherian, local ring and $O$ a discrete valuation ring (with $\overline{\omega}$ a generator of its maximal ideal) together with a surjective homomorphism $\lambda:A \rightarrow O$. (We denote $C_0$ the category of all such pairs $(A, \lambda))$ Let $\Phi_A:=ker(\lambda)/(ker(\lambda))^2$. I have a proof claiming that a certain a reduced Gorenstein ring of Krull dimension 1 is not a complete intersection ring, but the proof leaves out many details, so I would be grateful if someone could fill in those details: (1) How does he know that $\Phi_A \cong k^3$ (2) How does he work out the basis and the prime ideals of A (3) How does he know which one is the maximal one (4) What does he mean by "defining relations", how does he find the embedding dimension and how do these two imply that $A/(\overline{\omega})$ is not complete intersection (5) How does the ring being Cohen-Macaulay of dimension $1$ imply that it is reduced? (6) How does he find the socle of $A/(\overline{\omega})$, its dimension and that it is Gorenstein (7) How does this imply that $A$ itself is Gorenstein of dimension 1, but not complete intersection.
 Jbuck
Jbuck
Answer
- The questioner was satisfied with and accepted the answer, or
- The answer was evaluated as being 100% correct by the judge.
-
Hi, can you give me some feedback on the answer? I'm not sure if that's satisfying or you want more details, but I'm also rather busy these days so I'd avoid investing more time into it if it's not necessary. Thank you!
-
Hello there, would you be available to answer some more questions about fields and galois theory today?
-
I'm giving $60 for each
-
I should be available, yes.
-
Do you have an expected time for the questions?
-
Yes the deadline is about 3 hours
- answered
- 1425 views
- $70.00
Related Questions
- $Tor$ over finite rings
- Homomorphism
- Zariski Topology and Regular Functions on Algebraic Varieties in Affine Space
- Elementary group theory and number theory questions involving prime numbers, permutation groups and dihedral groups. Introduction to number theory questions
- Generating set for finitely generated submodule of finitely generated module
- Length of a matrix module
- Tensor Product II
- [Modules] Show that $h_3$ is injective given comutative diagram



This is not my area of expertise, but the offered bounty seems low for the level of the question.
I have a solid background in algebra, but not this branch in particular. I think I figured out a few details, but going through everything formally is a lot of work. I'm willing to take the question if we can sort of "work together" towards a solution, I'll write what I figured out and then you can tell me if you're satisfied with it or need more info. It is quite a lot of work though, so a slightly higher bounty would be appreciated. Would that work for you?
@Alessandro Iraci yes, if you can provide me with a solid backbone of details, I guess we can work the rest of them out. Keep in mind, you don't need to add details like, for example, "from the 1st isomorphism theorem we have that..." or "the kernel of the map being zero implies that the map is injective", taught in a standard undergraduate algebra module. I will increase the bounty as well.
Alright, I'll take it, I hope I can get enough insight to complete the proof.