How to show that the composition of two riemannian isometries is an isometry?
If for Riemannian charts $(P, g)$, $(Q, h)$ and $(R, i)$ I have two Riemannian isometries (differentiable bijection with differentiable inverse), one $\phi: (P, g) \to (Q,h)$ such that $g = \phi^{*}h$ and one $\rho: (Q,h) \to (R, i)$ such that $h = \rho^{*}i$, how do I show that the composition of these two isometries is again an isometry?
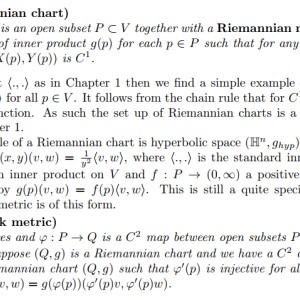
See the file/image for more information on the used notation here.
Answer
Answers can only be viewed under the following conditions:
- The questioner was satisfied with and accepted the answer, or
- The answer was evaluated as being 100% correct by the judge.
1 Attachment
Erdos
4.8K
-
Thank you Philip! It really helps a lot:). I will consider that indeed, it was my first time using this website so I was not sure what to expect
-
I am glad I was able to help.
The answer is accepted.
Join Matchmaticians Affiliate Marketing
Program to earn up to a 50% commission on every question that your affiliated users ask or answer.
- answered
- 840 views
- $5.00
Related Questions
- Analyzing Concave Down Segments of the Sinusoidal Curve
- Find the Curvature of Parabolic Wave
- Geometric Representation Problem
- Petra is organising a team building activity day with work colleagues . She is considering two options, which both offer packages for groups of up...
- Land area calculation/verification
- Get area of rotated polygon knowing all coordinates and angle.
- I am looking for a formula to calculate the radii of an ellipsoid from coordinates of non-coplanar locii on its surface.
- Find the volume of the solid obtained by rotating $y=x^2$ about y-axis, between $x=1$ and $x=2$, using the shell method.
