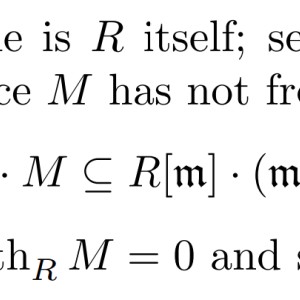Length of finitely generated module over $0$-dimensional Gorenstein local ring
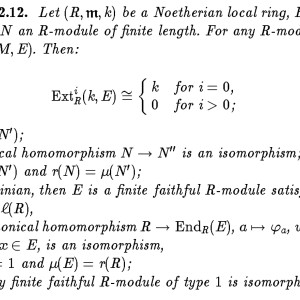
Please see attached images: Can someone slowly walk me through the details of the proof of Lemma 4.1 using basic commutative algebraic arguments and definitions?
1) Why $R[\mathfrak{m}] \cong k$? (where $k$ is the residue field of $R$)
2) I know length is additive on direct sums, but how is the RHS of the inequality additive as well, since it is a product?
3) Why does the inequality coincide when $R$ is the module over itself?
4) How does $R$ being Gorenstein of dimension $0$ imply that $R$ is the only indecomposable injective module, and why is its injective hull free as a result?
5) Why $M \subseteq \mathfrak{m}F$?
6) Why does $M=0$ imply the desired result?
Jbuck
152
Join Matchmaticians Affiliate Marketing
Program to earn up to a 50% commission on every question that your affiliated users ask or answer.
- closed
- 1572 views
- $44.00
Related Questions
- Reverse this equation/function (2d to isometric)
- Prove that $A - B=A\cap B^c$
- Mathematical Model: Discrete Logistic Growth and Fish Harvesting
- Tensor Product
- Free Body Diagram: determine the vertical reaction at the left hand beam support.
- Induced and restricted representation
- Certain isometry overfinite ring is product of isometries over each local factor
- Confused on this graph question, not sure how to reduce it to linear and It looks too wonky to draw a best fit line, probably won't take long
