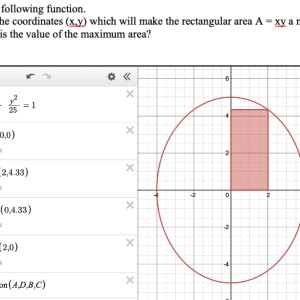
(a) Find the coordinates (x,y) which will make the rectangular area A = xy a maximum. (b) What is the value of the maximum area?
(a) Find the coordinates (x,y) which will make the rectangular area A = xy a maximum.
(b) What is the value of the maximum area?
 Jayellis00
Jayellis00
60
Answer
Answers can only be viewed under the following conditions:
- The questioner was satisfied with and accepted the answer, or
- The answer was evaluated as being 100% correct by the judge.
4.8K
-
Thanks for the tip!
The answer is accepted.
Join Matchmaticians Affiliate Marketing
Program to earn up to a 50% commission on every question that your affiliated users ask or answer.
- answered
- 1199 views
- $10.00
Related Questions
- Convergence integrals
- Find the limit as x-->0 for y = (e^x- 1)/[sin(nx)]
- Differentiate $f(x)=\int_{\sin x}^{2} \ln (\cos u) du$
- Evaluate$\int \sqrt{\tan x}dx$
- A telephone line hanging between two poles.
- Find $\lim\limits _{n\rightarrow \infty} n^2 \prod\limits_{k=1}^{n} (\frac{1}{k^2}+\frac{1}{n^2})^{\frac{1}{n}}$
- Find all functions $f: \mathbb{Z} \rightarrow \mathbb{Z}$ such that $f(2n)+2f(2m)=f(f(n+m))$, $\forall m,n\in \mathbb{Z}$
- Vector-valued functions and Jacobian matrix