Module isomorphism and length of tensor product.
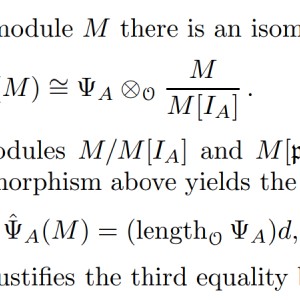
See image: Why is the isomorphism of $\Psi_A$ modules true and how do we show the length equality? Notation: $A$ is a commutative, Noetherian, local ring together with a surjective homomorphism to a discrete valuation ring: $\lambda: A \rightarrow \mathcal{O} , p_A=ker(\lambda), I_A= Ann[p_A]$ (the annihilator of the kernel of the map), $\Psi_A=\mathcal{O}/\lambda(I_A), M[I]=\{m \in M: mi=0, \forall i \in I\}$ (for every ideal $I$ of $A$), $\hat{\Psi}_A= \frac{M}{M[I_A]+I_AM}$. Please let me know if you need to know any more facts.
 Jbuck
Jbuck
152
Answer
Answers can only be viewed under the following conditions:
- The questioner was satisfied with and accepted the answer, or
- The answer was evaluated as being 100% correct by the judge.
 Dynkin
Dynkin
779
The answer is accepted.
Join Matchmaticians Affiliate Marketing
Program to earn up to a 50% commission on every question that your affiliated users ask or answer.
- answered
- 1339 views
- $30.00
Related Questions
- Internal Rate of Return vs Discount Rate
- Is the infinite series $\sum_{n=1}^{\infty}\frac{1}{n \ln n}$ convergent or divergent?
- Calculating Speed and Velocity
- Compounding interest of principal P, where a compounding withdrawal amount W get withdrawn from P before each compounding of P.
- There are a total of 95 coins, quarters and dimes, and the total is $15.35. How many dimes are there ?
- Certain isometry overfinite ring is product of isometries over each local factor
- Solve $abc=2(a-2)(b-2)(c-2)$ where $a,b $ and $c$ are integers
- Algebra question