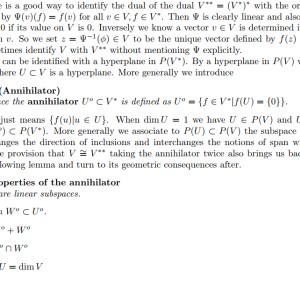
Three questions on the annihilator

The three questions are:
(a) Prove that if $ U ? V$ is a linear subspace then $U^\circ $ is also a linear subspace of $V^*$.
(b) If $f \in V^*$ is a non-zero element how is $(Span(f))^o $ related to $ker f$?
(c) When $V = R^3$,  describe the subspace  $(Span(\epsilon^2 + \epsilon^3))^\circ$.

More info on the annihilator is found in the image.
I think for question (b) you need to show that $\Psi(ker(f))=(Span(f))^\circ$ (and you possibly need the Lemma in the image as well).