Consider the plane in R^4 , calculate an orthonormal basis
[Orthogonal complement in dimension 4]. Consider the plane M in R^4 defined by the following equations:
(Image 1)
(a) Calculate an orthonormal basis (v1, v2) for M
(b) Calculate an orthonormal basis (W1, W2) for the orthogonal complement of M, N= M ⊥
(c) Prove that the family β = (V1, V2,W1,W2) is an orthonormal basis for R^4
 Vienrods
Vienrods
28
Answer
Answers can only be viewed under the following conditions:
- The questioner was satisfied with and accepted the answer, or
- The answer was evaluated as being 100% correct by the judge.
1.7K
The answer is accepted.
Join Matchmaticians Affiliate Marketing
Program to earn up to a 50% commission on every question that your affiliated users ask or answer.
- answered
- 1214 views
- $8.00
Related Questions
- Singular Value Decomposition Example
- Consider the function, prove that it's bilinear, symmetric, and positive definite
- [change of basis] Consider the family β = (1 + x + x 2 , x − x 2 , 2 + x 2 ) of the polynomial space of degree ≤ 2, R2[x].
- Find the eigenvalues of $\begin{pmatrix} -1 & 1 & 0 \\ 1 & 2 & 1 \\ 0 & 3 & -1 \end{pmatrix} $
- Let $\mathbb{C} ^{2} $ a complex vector space over $\mathbb{C} $ . Find a complex subspace unidimensional $M$ $\subset \mathbb{C} ^{2} $ such that $\mathbb{C} ^{2} \cap M =\left \{ 0 \right \} $
- Get area of rotated polygon knowing all coordinates and angle.
- Question about interest earned
- Relating dot product divided with square of the vector while changing basis of vector
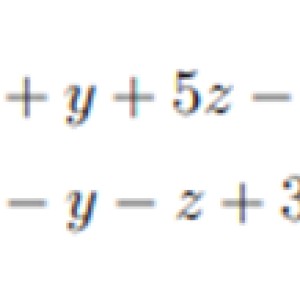

Do you need all the calculations or are you happy with the set of vectors (and the way to get them) and do the calculations yourself?
the set of vectors and how to get them is fine