Exercise on Ito's rule for two correlated stocks.
Answer
Answers can only be viewed under the following conditions:
- The questioner was satisfied with and accepted the answer, or
- The answer was evaluated as being 100% correct by the judge.
2 Attachments
Mathe
3.6K
The answer is accepted.
Join Matchmaticians Affiliate Marketing
Program to earn up to a 50% commission on every question that your affiliated users ask or answer.
- answered
- 600 views
- $25.00
Related Questions
- 3 Multi-step response questions
- Convergence of $\sum\limits_{n=1}^{\infty}(-1)^n\frac{n+2}{n^2+n+1}$
- Prove that $tan x +cot x=sec x csc x$
- Prove that $\int _0^{\infty} \frac{1}{1+x^{2n}}dx=\frac{\pi}{2n}\csc (\frac{\pi}{2n})$
- Find the antiderrivative of $\int \frac{v^2-v_o^2}{2\frac{K_e\frac{q_1q_2}{r^2}}{m} } dr$
- Please answer the attached question about Riemann integrals
- Show that the line integral $ \oint_C y z d x + x z d y + x y d z$ is zero along any closed contour C .
- Applied calc question 2 and 3
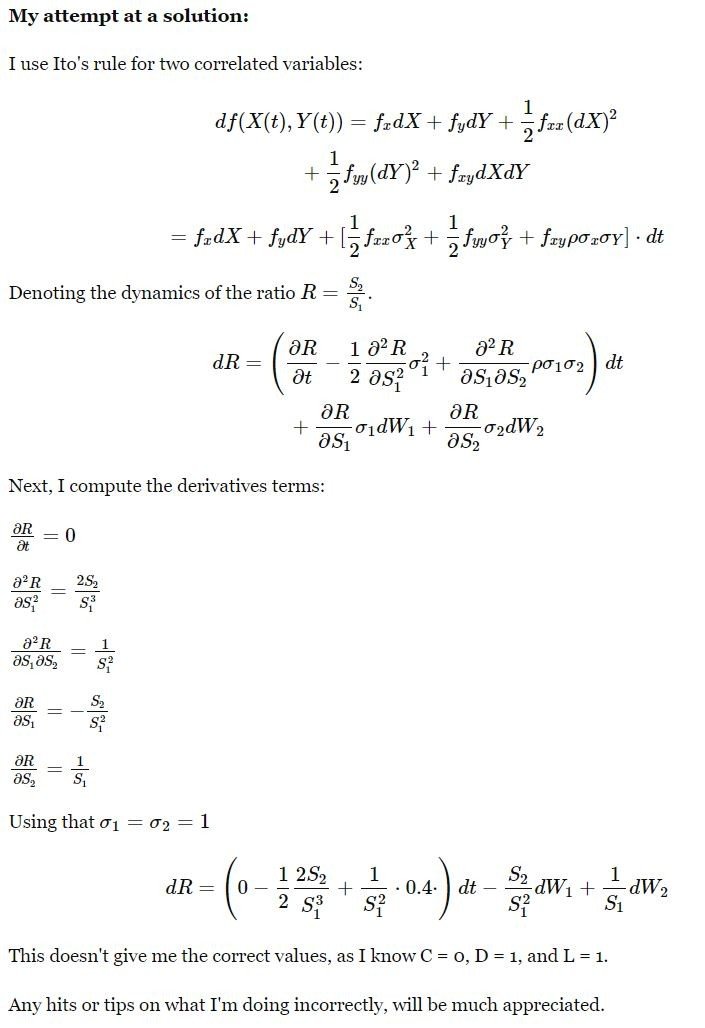
I believe a question in advanced math material warrants a much higher bounty.
What are they typically worth?
I believe for a problem on stochastic calculus and going over your solution, a bounty of $25 is warranted.
Thank you for making me aware :)
I think you set it to $20 and not $25. Perhaps that was on purpose.
That's the amount of credit I had left. Will see if it is enough.
Would it be enough to just point out the mistakes you have? Or do you want the correct derivation as well? Because I've seen two mistakes already.
The derivation doesn't have to be detailed, but would like a rough sketch and answers.
I've finished writing a response explaining the correct idea and listing the mistakes you made. I would submit it immediately for $25.
Thank you for your suggestions. It was my understanding that for Brownian motion, mu = 0 and sigma = 1. Thus, the terms I did not include are not needed. However, I still end up with the incorrect values - I might just be an idiot at calculating this, but could you provide the values of the parameters?
I will edit my reponse to address your comment. But bare in mind that S_i are not Brownian motions. W_i are brownian motions.
Increased it to 25 :) Looking forward to it
Thank you so much Mathe. Your explanation really helped me understand it. Have you received the money?
I did! Thank you!