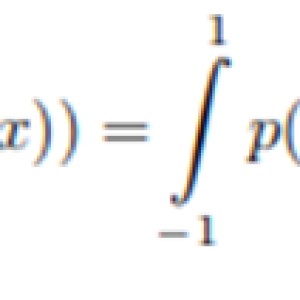Consider the function, prove that it's bilinear, symmetric, and positive definite
[Orthogonal Polynomials]. Consider the following bi-valued function defined on the space of polynomials of degree ≤ 2:
(Image 1)
For whichever polynomials p(x), q(x) ∈ P≤2. Consider the following polynomials:
p0(x) = 1, p1(x) = x, p2(x) = (3/2 )x² − 1/2 .
(a) Prove that F is bilinear, symmetric, and positive definite
(b) Prove that the family (p0, p1, p2) is an orthogonal basis of P≤2.
 Vienrods
Vienrods
28
Answer
Answers can only be viewed under the following conditions:
- The questioner was satisfied with and accepted the answer, or
- The answer was evaluated as being 100% correct by the judge.
1.7K
The answer is accepted.
Join Matchmaticians Affiliate Marketing
Program to earn up to a 50% commission on every question that your affiliated users ask or answer.
- answered
- 1194 views
- $8.00
Related Questions
- [change of basis] Consider the family β = (1 + x + x 2 , x − x 2 , 2 + x 2 ) of the polynomial space of degree ≤ 2, R2[x].
- Linear Algebra Assistance: Linear Combinations of Vectors
- Find the values of x
- [ eigenvalues and eigenvectors] Prove that (v1, v2, v3) is a basis of R^3
- Length of a matrix module
- Linear algebra
- Space of all matrices with given column space
- Hello! I Would like a proof detailed of the following question.