Help with linear algebra HW. Please show work!
Given that
$$\begin{vmatrix} a & b & c \\ 10d & 10e & 10e \\ g & h & i \end{vmatrix}=7,$$ find the value of
$$\begin{vmatrix} d & e & f \\ a-2g & b-2h & c-2i \\ 5g & 5h & 5i \end{vmatrix}. $$
Hint: Use row operations to transform first to
$$\begin{pmatrix} a & b & c \\ d & e & f \\ g & h& i \end{pmatrix}, $$ then continue to get the ending matrix, keeping track of how your operations change the determinant
Commander96
12
Answer
Answers can only be viewed under the following conditions:
- The questioner was satisfied with and accepted the answer, or
- The answer was evaluated as being 100% correct by the judge.
Daniel90
443
-
Let me know if you have any questions.
The answer is accepted.
Join Matchmaticians Affiliate Marketing
Program to earn up to a 50% commission on every question that your affiliated users ask or answer.
- answered
- 703 views
- $2.00
Related Questions
- General solutions of the system $X'=\begin{pmatrix} a & b \\ c & d \end{pmatrix} $
- Linear Algebra - Vectors and Matrices
- Let $H$ be the subset of all 3x3 matrices that satisfy $A^T$ = $-A$. Carefully prove that $H$ is a subspace of $M_{3x3} $ . Then find a basis for $H$.
- Find the null space of the matrix $\begin{pmatrix} 1 & 2 & -1 \\ 3 & -3 & 1 \end{pmatrix}$
- Find the values of a, for which the system is consistent. Give a geometric interpretation of the solution(s).
- Show that the $5\times 5$ matrix is not invertable
- two short Linear Algebra questions
- Numerical Linear Algebra Question
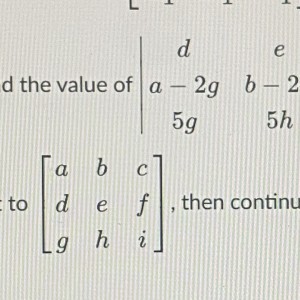
It's hard to read the formulas in your question. Why don't you post a picture of the question?
Didn’t realize I could do that. I just uploaded a picture.
I revised your question. The main change was that I used "pmatrix" in the LaTeX code.
And also vmatrix!