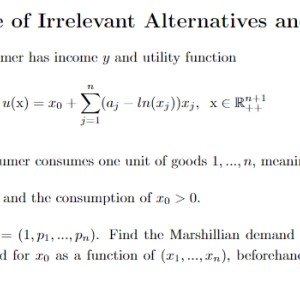
Math heavy microeconomics. Finding Marshillian demand (logit function)
Background: "In microeconomics, a consumer's Marshallian demand function is the quantity he/she demands of a particular good as a function of its price, his/her income, and the prices of other goods, a more technical exposition of the standard demand function. It is a solution to the utility maximization problem of how the consumer can maximize his/her utility for given income and prices"
Biggest issue is finding the demand beforehand for $x_{0} $ as a function of $x_{1},...x_{n}$. It should be obvious and trivial to find the demand beforehand, so I hope someone with knowledge in mathematical microeconomics theory can help me solve the problem.
The problem that is to be solved is attached as a picture.
My take on the problem however I don't see how this helps me find the demand beforehand for $x_{0} $ as a function of $x_{1},...x_{n}$ : Link to my take (since I can't add a picture in edit) https://imgur.com/a/6941Zh3
- closed
- 598 views
- $15.22
Related Questions
-
For the LP attached , suppose at least 8 oz of chocolate
and at least 9 oz of sugar are required (with other
requirements remaining the same). What is the new optimal
z-value? - Solve this problem using branch and bound algorithm.
- Optimization problem
- Find the extrema of $f(x,y)=x$ subject to the constraint $x^2+2y^2=2$
- Find the absolute extrema of $f(x,y) = x^2 - xy + y^2$ on $|x| + |y| \leq 1$.
- Optimization Quick Problem
- Urgency Can you help me Check these Applications of deritive.
- (Calculus 1) Basic Calc: Derivatives, optimization, linear approximation...
This is not my area of expertise, but you should offer enough bounty that worths someone's time with "someone with advanced knowledge in mathematical microeconomics theory" to spend time to write a good solution to your question. The bounty seems too low to me.
If the person has experience with these types of questions then they can answer this in less than 10 min.
I am not an economist, but it you can explain and write your question as an optimization problem, I should be able to help. This sounds like a constraint optimization problem to me.
Hi Phillip. I have tried solving it by optimizing it subject to the constraint, but I don't see how that helps me find the demand of x0 beforehand. There should be some obvious answer that can be seen instantly when looking at the utility function.
But you are correct it is an optimization problem. However I think a person with some experience in microeconomics is needed.
You don't seem to be enforcing x_1+x_2+... x_n=1.
Yeah thats right, and I think thats where I need someone with microeconomic intuition. Because maybe a transformation of the utility function to a Cobb Douglas or something could fix that problem as the exponent sums to 1 in those types of functions.
You probably need to use Lagrange-Multipliers.
Do you know how to optimize this problem while enforcing sum of x_j's = 1 and while ensuring that the use of x_0 is positive?
I may be. My suggestion is that you write your question as an optimization problem with clearly stated constraints and objective functions (most users here have mathematical background). Offer a good bounty, and you will most likely get an answer. Also note that this is not a 10 minute problem. I have spent more than 15 minutes writing these comments!
It takes time and effort to become experienced in microeconomics to solve this problem in '10 minutes'.