Geometry without using trigonometry
So I'm supposed to find the area of the blue figure without using trigonometry. What do I have to do to find this?
Noobis
Answer
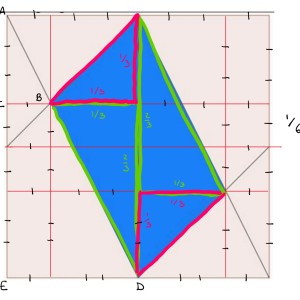
Look at the attached picture. We have that the triangles $ABC$ and $ADE$ are similar, so since $AE = 2AD$ then $AC = 2AB$. Now since there are so many right angles, all the segments marked with a single tick have the same length, and the ones marked with two ticks have twice that length. It follows that, if the big square has side lenght equal to $1$, then $AB = 1/6$. Now we can split the blue figure in four triangles, whose areas are easy to calculate: the green ones have area $1/9$, the pink ones $1/18$. Putting all those together, the area of the blue figure is \[ 2 \cdot \frac{1}{ 9} + 2 \cdot \frac{1}{18} = \frac{3}{9} = \frac{1}{3}. \]
Alessandro Iraci
-
I obviously meant BC instead of AB and DE instead of AD, but I guess you figured it out!
- answered
- 2478 views
- $5.89
Related Questions
- Determine the surface area of a ball, rotating a function about $x$-axis.
- Thickness of Multiple Spherical Shells
- Probability that the distance between two points on the sides of a square is larger than the length of the sides
- How to show that the composition of two riemannian isometries is an isometry?
- Pulley System
- How to recalculate 2D polygon side lengths when tilt is applied in 3D space?
- Why if $\frac{opp}{adj} =x$, then $x \times hyp =$ The length of a line perpendicular to the hypotenuse with the same height.
-
Find a general solution for the lengths of the sides of the rectangular parallelepiped with the
largest volume that can be inscribed in the following ellipsoid
Low bounty! Your deadline is also too short.
I think there's some missing info? Do the diagonal lines pass through the middle points of the edges of the square?