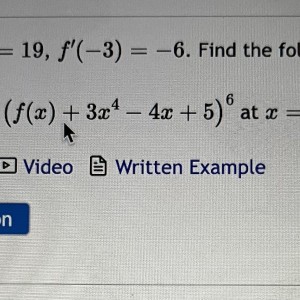
Derivatives again. Thank you!
Answer
Answers can only be viewed under the following conditions:
- The questioner was satisfied with and accepted the answer, or
- The answer was evaluated as being 100% correct by the judge.
Savionf
573
-
Both were wrong. I get one more try
-
A minus sign was missing in the final answer. Please try again. It should work now.
-
-
It didn’t work. The answer was 6(19+260)^5(-6-328). I ran out of fund, I’ll try to redo a different one on my own
-
My answer is the same as 6(19+260)^5(-6-328). Probably it is did not accept it because of the format of the answer. 6(19+260)^5(-6-328)=6(279)^5(-334), same as my answer.
-
The answer is accepted.
Join Matchmaticians Affiliate Marketing
Program to earn up to a 50% commission on every question that your affiliated users ask or answer.
- answered
- 682 views
- $3.00
Related Questions
- Spot my mistake and fix it so that it matches with the correct answer. The problem is calculus based.
- Is $\int_1^{\infty}\frac{x+\sqrt{x}+\sin x}{x^2-x+1}dx$ convergent?
- Generalization of the Banach fixed point theorem
- Prove the trig identity $\sec x- \sin x \tan x =\frac{1}{\sec x}$
- calculus question
- Find the limit as x-->0 for y = (e^x- 1)/[sin(nx)]
- Two persons with the same number of acquaintance in a party
- Integral of $\arctan x$
