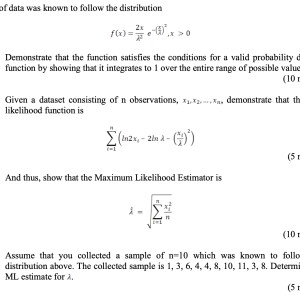
Show that the MLE for $\sum_{i=1}^{n}\left(\ln{2x_i} - 2\ln{\lambda} - \left(\frac{x_i}{\lambda}\right)^2\right)$ is $\hat{\lambda} = \sqrt{\sum_{i=1}^{n}\frac{x_i^2}{n}}$.
Firstly, as my assignment is due in 2 hours, I am offering $30. The due time for this post is set to 1 hour only - for the sake of speed, feel free to use pen/pencil on pape
Hi, I have solved the previous question (b), which was:
Consider the function $f(x) = \frac{2x}{\lambda^2}e^{-(\frac{x}{\lambda})^2}$.
Given a dataset consisting of $n$ observations, $x_1, x_2, ..., x_n$, demonstrate that the log-likelihood function is $\sum_{i=1}^{n}\left(\ln{2x_i} - 2\ln{\lambda} - \left(\frac{x_i}{\lambda}\right)^2\right)$.
My solution to (b) is attached as a screenshot.
Now, for (c), they want me to find the MLE for the summation expression from (b) - it is the question I have put in the title of this post.
Please show clear workings and do not skip any steps - this is for a graded college assignment. Thank you.
Angmingwen
Answer
- The questioner was satisfied with and accepted the answer, or
- The answer was evaluated as being 100% correct by the judge.
1 Attachment
Mathe
-
Sorry Mathe, I must say I'm a bit confused - I don't follow the first 3 lines. Where does 2n*ln(lambda) come from in the 2nd line? How did a summation operator in the last expression on the 2nd line? Why are there 2 summation operators on the 3rd line?
-
I hope you provide more explanation. This isn't just for you to solve, but also for me to understand ~ I will need to transfer it into my own work.
-
I'll be more explicit next time!
-
-
Nevermind, its quite basic. Thanks, I will accept it now.
- answered
- 386 views
- $30.00
Related Questions
- Improper integral
- Question for KAV1
-
Limit graphs
- Differentiate $f(x)=\int_{\sqrt{x}}^{\sin^2 x}\arctan (1+e^{t^2})dt$
- Optimization problem
- Find $\lim_{x \rightarrow} x^2 \sin(1/x) $. Cite theorems used and show all work
- Volume of the solid of revolution for $f(x)=\sin x$
- Improper integral convergence
I can't find the screenshot.
Sorry Mathe. Just attached it.
That was my working for the previous question.
The screenshot lacks one piece of context - the function $f(x)$ is given in the question. It is not included in the screenshot.
I added a second file. This file contains the entire question and all its subquestions. I solved (1), and (2), and I asked for (3) on this platform.
MLE being Maximum Likelihood Estimator.
Please let me know if you need anything else - also, my hard deadline for the assignment is exactly in 2.5 hours, so, if you need more time, I will extend to 2 hours. However, the earlier obviously the better for me, so I have more time to understand and transfer it onto Latex.
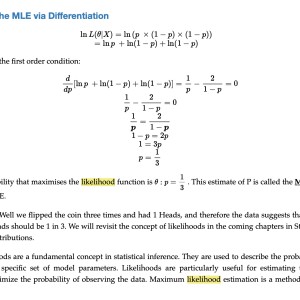
The 3rd file is an example of MLE being used to find the probability of a coin getting heads when flipped 3 times. Only problem is, I don't know how to apply the same concept\differentiation to a sum.
One takes derivative with respect to the parameter one wants to estimate, in this case, lambda. We can ignore any term that doesn't have any lambda, and treat any x_i observation as a constant.
Yeah sure I understand that!