Scalar fields, potentia

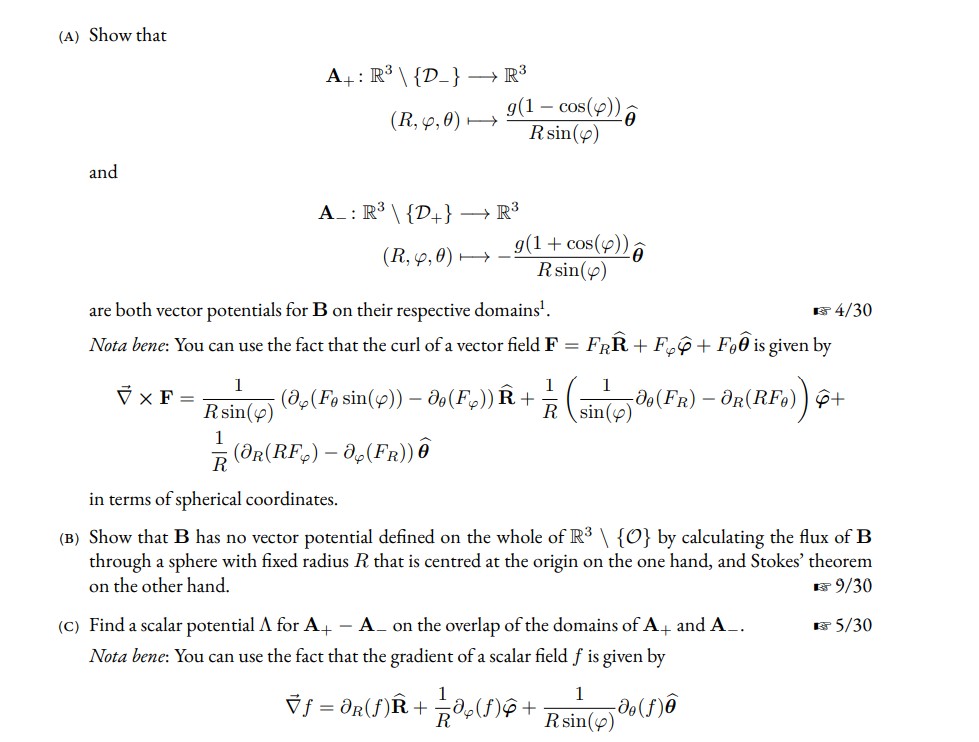
A) Let $F=\frac{g(1-\cos \varphi)}{R\sin \varphi}\hat{\theta}$. Note that $F_\theta=\frac{g(1-\cos \varphi)}{R\sin \varphi}$, $F_\varphi=0$, and $F_{R}=0.$

Note also that 
\[\lim_{\varphi \rightarrow 0}\frac{g(1-\cos \varphi)}{R\sin \varphi}=\lim_{\varphi \rightarrow 0}\frac{g\sin \varphi}{R \cos\varphi}=0.\]
So $F$ is continuous on $\varphi =0$, which is indeed $\mathcal{D}_+.$

\[\nabla \times F=\frac{1}{R\sin \varphi}(\partial_{\varphi}(F_\theta \sin (\varphi))-\partial_\theta F_\varphi)\hat{R}+\frac{1}{R}\left( \frac{1}{\sin \varphi}\partial_\theta(F_R)-\partial_R(RF_\theta)\right)\hat{\varphi}+\frac{1}{R}\left( \partial_R(R F\varphi)-\partial_{\varphi}(F_R)\right)\hat{\theta}\]
\[=\frac{1}{R\sin \varphi}(\partial_{\varphi}(\frac{g(1-\cos \varphi)}{R})-0)\hat{R}+\frac{1}{R}\left( \frac{1}{\sin \varphi}\times 0-\partial_R(\frac{g(1-\cos \varphi)}{\sin \varphi})\right)\hat{\varphi}+\frac{1}{R}\left( 0-0\right)\hat{\theta}\]
\[=\frac{1}{R\sin \varphi}(g\frac{\sin \varphi}{R})\hat{R}+0\hat{\varphi}+0 \hat{\theta}=\frac{g}{R^2}\hat{R}=B. \]

Similarly, if we let  $F=-\frac{g(1+\cos \varphi)}{R\sin \varphi}\hat{\theta}$. Note that $F_\theta=-\frac{g(1+\cos \varphi)}{R\sin \varphi}$, $F_\varphi=0$, and $F_{R}=0.$

Note also that 
\[\lim_{\varphi \rightarrow \pi}\frac{-g(1+\cos \varphi)}{R\sin \varphi}=\lim_{\varphi \rightarrow \pi}\frac{g\sin \varphi}{R \cos\varphi}=0.\]
So $F$ is continuous on $\varphi =\pi$, which is indeed $\mathcal{D}_-.$

Also 

\[\nabla \times F=\frac{1}{R\sin \varphi}(\partial_{\varphi}(F_\theta \sin (\varphi))-\partial_\theta F_\varphi)\hat{R}+\frac{1}{R}\left( \frac{1}{\sin \varphi}\partial_\theta(F_R)-\partial_R(RF_\theta)\right)\hat{\varphi}+\frac{1}{R}\left( \partial_R(R F\varphi)-\partial_{\varphi}(F_R)\right)\hat{\theta}\]
\[=\frac{1}{R\sin \varphi}(\partial_{\varphi}(-\frac{g(1+\cos \varphi)}{R})-0)\hat{R}+\frac{1}{R}\left( \frac{1}{\sin \varphi}\times 0-\partial_R(\frac{g(1-\cos \varphi)}{\sin \varphi})\right)\hat{\varphi}+\frac{1}{R}\left( 0-0\right)\hat{\theta}\]
\[=\frac{1}{R\sin \varphi}(g\frac{\sin \varphi}{R})\hat{R}+0\hat{\varphi}+0 \hat{\theta}=\frac{g}{R^2}\hat{R}=B. \]

B) Suppose $B=\frac{g}{R^2}\hat{R}=\nabla \times F$, for some vector field $F$ that is defined on $\mathbb{R}^3 \backslash \{\mathcal{O}\}$.  Let $S$ be the sphere of radius  $R_0>0$. Then 

$$\iint\limits_S (\nabla \times \mathbf{F}) \cdot d\mathbf{S}=\iint\limits_S B\cdot dS=\iint\limits_S \frac{g}{R_0^2}ds=4\pi^2 \frac{g}{R_0^2}.$$
On the other hand using the Stokes' Theorm 
$$ \iint\limits_S (\nabla \times \mathbf{F}) \cdot d\mathbf{S}=\oint\limits_{\partial S} \mathbf{F} \cdot d\mathbf{r}=0 $$
and $\partial S= \emptyset$ (empty set). Hence we get $4\pi^2 \frac{g}{R_0^2}=0$ which is impossible. Thus $B$ has no vector potential defined on $\mathbb{R}^3 \backslash \{\mathcal{O}\}$.

C)  First note that 
\[A_{+}-A_{-}=\frac{g(1-\cos \varphi)}{R\sin \varphi}\hat{\theta}+\frac{g(1+\cos \varphi)}{R\sin \varphi}\hat{\theta}=\frac{2g}{R\sin \varphi}\hat{\theta}.\]
We want to find $f$ such that 
\[\nabla f=\partial_R(f)\hat{R}+\frac{1}{R}\partial \varphi(f)\hat{\varphi}+\frac{1}{R \sin \varphi} \partial_{\theta}(f)\hat{\theta}=\frac{2g}{R\sin \varphi}\hat{\theta}.\]
Hence 
\[\frac{1}{R \sin \varphi} \partial_{\theta}(f)=\frac{2g}{R\sin \varphi} \Rightarrow \partial_{\theta}(f)=2g\]
\[\Rightarrow f=2g\theta.\]