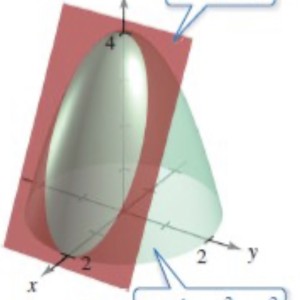
Get the volume and surface area of the paraboloid $z=4-x^2-y^2$ cut by the plane $z=4-2x$
Get the volume and surface area of the paraboloid $z=4-x^2-y^2$ cut by the plane $z=4-2x$
Please explain the process and the reasoning behind the steps made (not necessarily everything, just the most important steps)
8
Answer
Answers can only be viewed under the following conditions:
- The questioner was satisfied with and accepted the answer, or
- The answer was evaluated as being 100% correct by the judge.
1 Attachment
 Naturelover
Naturelover
88
-
Thanks for the answer! It’s pretty good explained (^_^)/‘
-
Of course. Thank you.
-
The answer is accepted.
Join Matchmaticians Affiliate Marketing
Program to earn up to a 50% commission on every question that your affiliated users ask or answer.
- answered
- 1728 views
- $10.00
Related Questions
- Use Stokes’ Theorem to calculate $\iint_{S} \nabla \times V· dS$ on the given paraboloid
- Applications of Stokes' Theorem
- Plot real and imaginary part, modulus, phase and imaginary plane for a CFT transform given by equation on f from -4Hz to 4Hz
- Create a function whose derivate is:
- Application of Integrals
- Volume of the solid of revolution for $f(x)=\sin x$
- Work problem involving pumping water from tank
- Answer is done but need help

Low bounty!
I doubled it. I hope it is better now.