Probability Question
(See picture for better formatting)
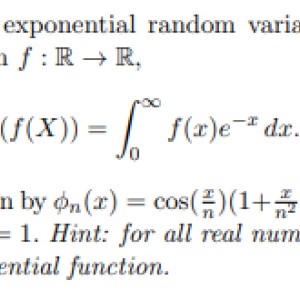
Let X be a standard exponential random variable, so for any non-negative Borel function f : R → R,
E(f(X)) = $\int_{0}^{\infty } f(x)\cdot e^{-x} dx$. Let φn : R → R be given by φn(x) = $\cos(\frac{x}{n}) \cdot(1+\frac{x}{n^2})^n$ for all x ∈ R. Show that lim n→∞ E(φn(X)) = 1.
Hint: for all real numbers x, $1 + x $ ≤ $e^x$ by convexity of the exponential function.
L Ellis
Answer
- The questioner was satisfied with and accepted the answer, or
- The answer was evaluated as being 100% correct by the judge.
1 Attachment
Erdos
-
You should set later deadlines for your questions. A few hours is too early. Ideally you wanna give at least 24 hours if possible.
-
I agree, but I'm in a bit of a crunch unfortunately!
-
I am busy at the moment, but If you extend the deadline of your other question I may be able to help. You may also want to offer higher bounties to give users more incentive to accept.
-
I can't extend unfortunately, the deadline is quite soon
-
Thanks for the rest, though!
- answered
- 847 views
- $8.00
Related Questions
- Calculating Driveway Gravel Area and Optimizing Cardboard Box Volume
- Prove that $\frac{d \lambda}{d \mu} = \frac{d \lambda}{d \nu} \frac{d \nu}{d \mu}$ for $\sigma$-finite measures $\mu,\nu, \lambda$.
- Generalization of the Banach fixed point theorem
- Show that the line integral $ \oint_C y z d x + x z d y + x y d z$ is zero along any closed contour C .
- Goalscorer Probability question
- Promotional Concept (Probability)
- Wiener process probability
- Integration headache, please help.
