Write a Proof
Hey! I would like assistance in writing a proof (step by step) to show the distance between a vertex and a co-vertex is the SAME as the distance between the center and a focus for hyperbolas. In simpler terms, why is x=y? I would really appreciate an illustration to go with the answer of some sort, and will pay however much is needed for the best possible answer.
Good Luck!
Answer
Answers can only be viewed under the following conditions:
- The questioner was satisfied with and accepted the answer, or
- The answer was evaluated as being 100% correct by the judge.
 Aman R
Aman R
649
The answer is accepted.
Join Matchmaticians Affiliate Marketing
Program to earn up to a 50% commission on every question that your affiliated users ask or answer.
- answered
- 1499 views
- $15.00
Related Questions
- Find $n$ such that $\lim _{x \rightarrow \infty} \frac{1}{x} \ln (\frac{e^{x}+e^{2x}+\dots e^{nx}}{n})=9$
- Compute the curl of $F=(x^2-\sin (xy), z-cox(y), e^{xy} )$
- Qaudratic Eqaution Graphing
- Explain partial derivatives v3
- Explain parameter elimination for complex curves v2
- Does $\lim_{n \rightarrow \infty} \frac{2^{n^2}}{n!}$ exist?
-
Limit graphs
- Calculus 1
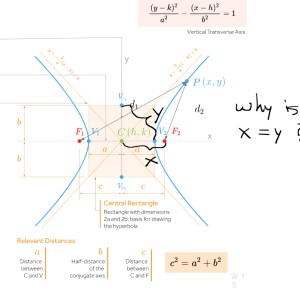

Can you increase it :) I will show a proof ! Thanks
Ok!
Sorry it looks like I cant add to a question's total bounty if it has already been accepted to be answered.
thank you so much!!
Welcome, it would be great if you accept the answer if you are satisfied. Thanks
Oops! My bad first time using the site
thank you so much