Help with 2 PDE questions

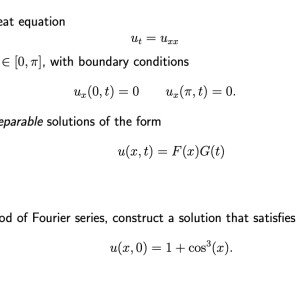
1. i) Let $y=x-t$ and $z=x+t$ then $$ u_{zy} =(u_{z})_{y}=(u_{x}+u_{t})_{y}=u_{xy}+u_{ty}  =u_{xx}-u_{xt}+u_{xt}-u_{tt}=u_{xx}-u_{tt}=0 $$ So we have \[ (u_{z})_{y}=0\implies u_{z}=F(z)\implies u=\int F(z)dz+C(y) \] Note that the constant of integration with respect to $z$ can depend on $y$, because when we integrate with respect to $z$ we are assuming that $y$ is fixed. Thus $u$ is the sum of a function of $z$ and a function of $y$. In other words \[ u(x,t)=A(x+t)+C(x-t) \] which implies \[ u_{t}(x,t)=A'(x+t)-C'(x-t) \] Now to find the functions $A,C$ we set $t=0$. Then we get $$ f(x) =u(x,0)=A(x)+C(x)\\ g(x)  =u_{t}(x,0)=A'(x)-C'(x)\\  \implies\int_{0}^{x}g(s)ds=A(x)-C(x)$$ Thus $A(x)=\frac{1}{2}f(x)+\frac{1}{2}\int_{0}^{x}g(s)ds$ and $C(x)=\frac{1}{2}f(x)-\frac{1}{2}\int_{0}^{x}g(s)ds$. So $$ u(x,t)  =A(x+t)+C(x-t)\\  =\frac{1}{2}(f(x+t)+f(x-t))+\frac{1}{2}\int_{x-t}^{x+t}g(s)ds $$ ii) By the above formula we have \[ u(x,\frac{1}{2})=\frac{1}{2}(f(x+\frac{1}{2})+f(x-\frac{1}{2}))=\begin{cases} 0 & |x|<\frac{3}{2}\\ \frac{1}{2} & \frac{3}{2}\le|x|<\frac{5}{2}\\ 1 & |x|\ge\frac{5}{2} \end{cases} \] \[ u(x,1)=\frac{1}{2}(f(x+1)+f(x-1))=\begin{cases} 0 & |x|<1\\ \frac{1}{2} & 1\le|x|<3\\ 1 & |x|\ge3 \end{cases} \] \[ u(x,2)=\frac{1}{2}(f(x+2)+f(x-2))=\begin{cases} \frac{1}{2} & |x|<4\\ 1 & |x|\ge4 \end{cases} \] which are easy to sketch (unfortunately there is no sketching tool in this environment, so I cannot plot them). 2. i) Suppose $u(x,t)=F(x)G(t)$ then $u_{xx}=F''G$ and $u_{t}=FG'$. So we must have \[ FG'=F''G\implies\frac{G'(t)}{G(t)}=\frac{F''(x)}{F(x)} \] Since one side is only a function of $t$ and the other side a function of $x$, both sides must be (the same) constant. Suppose \[ \frac{G'(t)}{G(t)}=\frac{F''(x)}{F(x)}=-\lambda^{2}\implies G'=-\lambda^{2}G,\;F''=-\lambda^{2}F \] Hence $G=Ce^{-\lambda^{2}t}$ and $F(x)=A\sin(\lambda x)+B\cos(\lambda x)$. But we know that $u_{x}=F'G=0$ at $x=0,\pi$. So $F'(0)=F'(\pi)=0$. But $F'=\lambda A\cos(\lambda x)-\lambda B\sin(\lambda x)$. So we must have $A=0$ to get $F'(0)=0$. And then $F'(\pi)=0$ implies that $\sin(\lambda\pi)=0$, which is true only if $\lambda=n$ is an integer. (We only consider positive integers since $\sin$ is an odd function and therefore negative integers will not give us new functions.) Finally we have the family of separable solutions \[ u_{n}(x,t)=C_{n}e^{-n^{2}t}\sin(nx) \] ii) Consider the general solution \[ u(x,t)=\sum_{n=1}^{\infty}u_{n}(x,t)=\sum_{n=1}^{\infty}C_{n}e^{-n^{2}t}\sin(nx) \] We have to choose the coefficients $C_{n}$ so that the initial condition is satisfied, i.e. \[ \sum_{n=1}^{\infty}C_{n}\sin(nx)=u(x,0)=1+\cos^{3}(x) \] So $C_{n}$ must be the Fourier sine coefficients of the function $1+\cos^{3}(x)$. Hence we have $$ C_{n}  =\frac{2}{\pi}\int_{0}^{\pi}(1+\cos^{3}(x))\sin(nx)\,dx\\  =\frac{2}{\pi}\int_{0}^{\pi}(1+\frac{3}{4}\cos(x)+\frac{1}{4}\cos(3x))\sin(nx)\,dx\\  =\frac{2}{\pi}\int_{0}^{\pi}\sin(nx)+\frac{3}{8}\sin((n+1)x)+\frac{3}{8}\sin((n-1)x)\\  \qquad+\frac{1}{8}\sin((n+3)x)+\frac{1}{8}\sin((n-3)x)\,dx\\  =\frac{2}{\pi}(\frac{-1}{n}\cos(nx)-\frac{3}{8n+8}\cos((n+1)x)-\frac{3}{8n-8}\cos((n-1)x)\\  \qquad-\frac{1}{8n+24}\cos((n+3)x)-\frac{1}{8n-24}\cos((n-3)x))|_{0}^{\pi}\\  =\frac{2}{\pi}(-\frac{(-1)^{n}-1}{n}-3\frac{(-1)^{n+1}-1}{8n+8}-3\frac{(-1)^{n+1}-1}{8n-8}\\  \qquad-\frac{(-1)^{n+1}-1}{8n+24}-\frac{(-1)^{n+1}-1}{8n-24}) $$