Discrete Math
1) Let $T$ be a tree with 47 vertices with the property that removing a vertex (and its adjacent edges) from $T $ creates two coherent components $T_1,T_2$ which are also trees. For$ i=1,2,$ we denote by Vi the number of vertices of Ti and by Ei the number of edges of $T_i$ . If $|V1|=|E2|+7$ find $|V1|$.
2) From image 1,2 find if they are Eulerian and Hamiltonian Graphs for each of them.
3) Consider the graph G with vertices $\{2,3,6,7,9,10,11,22\}$ and edges the pairs ${i,j}$ for which greatest_common_divisor$(i,j)≠1$
How many coherent components does G have? (Hint: draw the graph and measure the coherent components).
Jimisss
104
Answer
Answers can only be viewed under the following conditions:
- The questioner was satisfied with and accepted the answer, or
- The answer was evaluated as being 100% correct by the judge.
1 Attachment
Kav10
2.1K
The answer is accepted.
Join Matchmaticians Affiliate Marketing
Program to earn up to a 50% commission on every question that your affiliated users ask or answer.
- answered
- 915 views
- $30.00
Related Questions
- Combinatorics/counting: How many configurations are possible for m differenct objects in n boxes of unlimited occupany (m<n)
- Solve summation problem: $\sum_{k=1}^{n} \tfrac{2k+1}{k^{2}(k+1)^2 } $
- Combinatorics Questions: Card Pairs, Letter Arrangements, and Number Sequences
- How many balanced lists of n left and n right parentheses are there?
- Find the chromatic number of Kn, Kn,m, Cn.
- Count the number of decks of cards, where no king is on top of the ace of the same suit.
- [Discrete Mathematics] For {1,3,6,10,15,…}. Find a recursive formula.
- Logic Questions (𝐴→𝐶)∧(𝐵→𝐶)⊢(𝐴∧𝐵)→𝐶
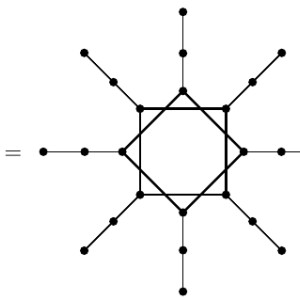
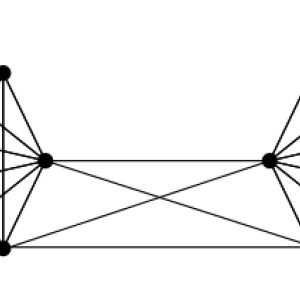
What makes a component "coherent"? Is that a synonym for "connected"?
coherent means connected yes. Thats the meaning