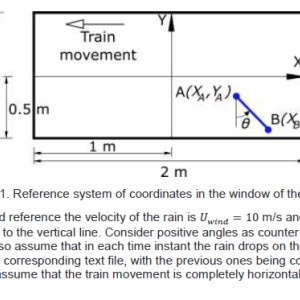
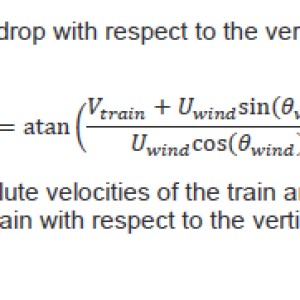
Obtaining the absolute velocity of a moving train based on angle of raindrops with respect to vertical axis
The problem is in the files attached.
I have been given the coordinates of the raindrops both start and end.
My question is how do I obtain the angle, and after that how do I arrange the equation to get Vtrain.
 Ice578
Ice578
Answer
- The questioner was satisfied with and accepted the answer, or
- The answer was evaluated as being 100% correct by the judge.
-
Leave a comment if you need any clarifications.
-
Thank you Philip, is ThetaWind always 30 degrees? I still dont quite get how to obtain the angle from the coordinates I was given. However everything you explained makes sense now.
-
In general ThetaWind may not be 30 degrees, but in your problem it is assumed to be 30 degrees.
-
Oh okay, so if I understand the assignment the first step in finding Vtrain should be to find the angle of the raindrop vectors using the coordinates that I was given? Should I do that using the slope?
-
Yes.
-
Great, thank you for the help Philip :),
-
Sorry for asking again Philip, but how would I obtain Vtrain with respect to a fixed refrence, so the absolute velocity of the train?
- answered
- 1527 views
- $4.92
Related Questions
- < Derivative of a periodic function.
- Explain what the problem means in laymens terms.
- (Calculus 1) Basic Calc: Derivatives, optimization, linear approximation...
- Similar shapes
- A generator at a regional Power Station produces an alternating voltage, according to the function, 𝑉 below:
- A telephone line hanging between two poles.
- Evaluate $\int_C (2x^3-y^3)dx+(x^3+y^3)dy$, where $C$ is the unit circle.
- Find a general solution for $\int at^ne^{bt}dt$, where $n$ is any integer, and $a$ and $b$ are real constants.